INTRODUCTION

Rheological measurements help us describe the complicated behavior of materials such as gels, polymer solutions and suspensions. The question “Is this material a solid or a liquid?” has no simple response. The answer lies in how much of the energy applied to make the material flow is elastically stored and how much is lost by viscous dissipation. This duality in the material response results in a wide spectrum of characteristics, where systems with similar shear viscosity may exhibit significantly different extensional viscosity.

In order to predict the process conditions that will optimize fluid flow or to characterize the final product properties, a thorough rheological characterization of materials in both shear and extensional flow conditions is recommended. e-VROC^® technology provides R&D and QC scientists with a valuable tool to characterize and categorize elasticity of viscoelastic fluids. Applications include fiber spinning, roll coating, blow molding, inkjet printing, film drawing and flow through porous media.

In this study we present measurements of apparent extensional and shear viscosities for two non-Newtonian shear thinning fluids (0.5 % water solution of Xanthan gum and 0.5% PVDF in MNP) and one Newtonian mineral standard oil N35 Lot. 14301 (Cannon Instruments). Combining the shear and extensional data allows us to exclude shear from the extensional flow and provide a better characterization of the materials response to planar extension.

PRINCIPLE AND TECHNOLOGY

Extensional viscosity is a measurement of the resistance of a fluid to stretch. Extensional flows result in a strong orientation of polymer molecules and asymmetric particles. Most industrial processes present mixed flows involving shear and extension. The resistance to flow, or viscosity, is affected by the orientation, and this has significant implications not only on the design and tuning of manufacturing processes but also on the final product properties. Therefore, understanding of both extensional and shear viscosity is essential for precise process control.

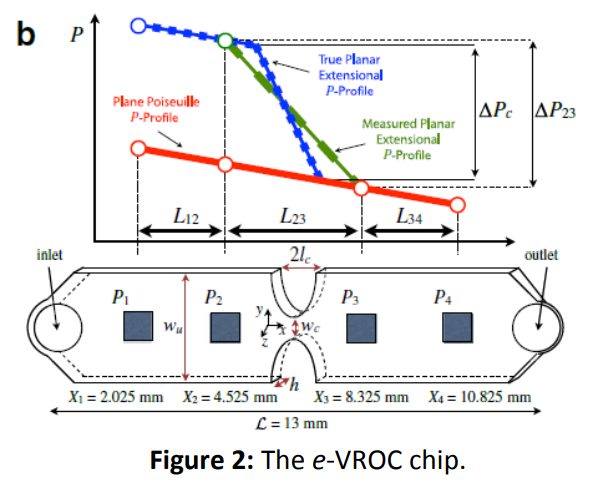
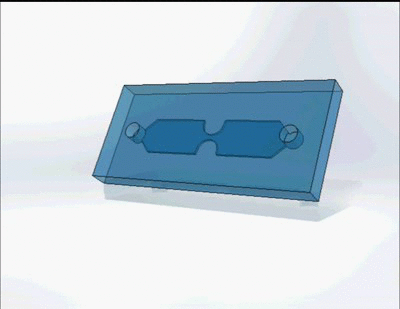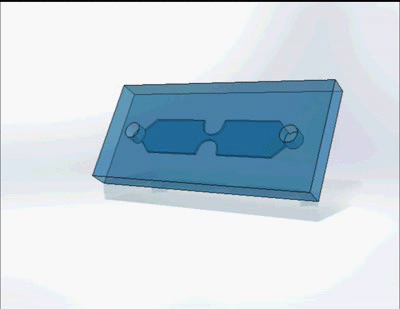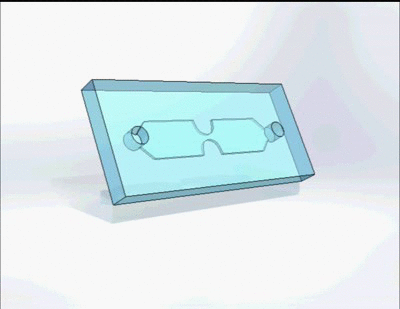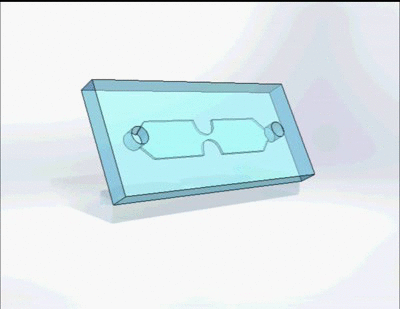
Figure 1. Pressure profiles inside flow channel (top). Schematic of e-VROC® cell (bottom) [1].

e-VROC^® chip operation principle is exemplified in Fig. 1. The fluid under study is subjected to flow in a microfluidic channel with a hyperbolic constriction at its center. The pressure drop through the channel is monitored by a MEMS pressure sensor array at the base of the channel. Once the pressure drop through the constriction ΔP_c  is obtained, the apparent extensional viscosity η_E,c is calculated as:

where ε_H  is the Hencky strain and the extensional strain rate. An extensive review of the causes of the deviation from true extensional viscosity is given by Ober et al. [1]. Here we focus on the experimental protocol to exclude the shear contribution due to shearing near the walls. In order to do so, we will assume a power law constitutive equation for the dependence of shear viscosity on shear rate.

CORRECTION PROTOCOL

The correction protocol proposed herein consists of three simple steps:

1. Establish power law parameters m and n corresponding to the shear viscosity behavior of the sample.

2. Obtain the viscous pressure drop ΔP_c,v due to shearing at the wall using Eqns. A1 or A2 and subtract it from the measured pressure drop at the constriction ΔP_c to obtain the pressure drop caused by the fluid resistance to stretching ΔP_{c, e.}

3. Recalculate extensional viscosity according to:

   

Shear Viscosity

Shear viscosity measurements were obtained with m-VROC^®, which is ideal for R&D applications where sample size, automation, and a wide dynamic range of viscosities and shear rates are important. For this kind of application accuracy and repeatability are a necessity [2].

True shear viscosity versus true shear rate results are presented in Fig. 2. Both Xanthan and PVDF solution show non-Newtonian (shear thinning) behavior that is well characterized by a power law over a wide range of shear rates. m-VROC^® is a powerful tool for characterizing the non-Newtonian behavior of complex fluids. Figure 2 shows how 0.5% Xanthan has a stronger shear thinning behavior than 0.5% PVDF. The results of fitting a power law to experimental data are summarized in Table 1. For comparison purposes we have included results for N35 standard oil in Fig. 2 and Table 1.

Table 1. Power law parameters η = mý^n-1

	Fluid		Newtonian		m [mPa-s]		η
0.5% Xanthan		No		3238.5		0.215
0.5% PVDF		No		375.44		0.796
N35		Yes		51.79		1

 

Figure 2. Shear viscosities versus shear rates. Solid lines represent the power law fits. Dashed line (Ref.) represents the certified reference viscosity for N35.

Extensional Viscosity

The pressure drop through the channel constriction is measured as a function of the extensional strain rate using e-VROC^®. Viscous and extensional pressure drops are calculated using Eqns. A1 – A3 from the appendix. The three different pressure drops are included in Fig. 3.

Figure 3. Pressure drop measured in the constriction (black) with extensional (blue) and shear (red) components for 0.5% Xanthan gum. Note that ΔP_{c =} ΔP_{c, e +} ΔP_{c, v.}

_{e-VROC^® system provides measurements of apparent extensional viscosity based on the pressure drop through the constriction using Eq. (1). Using the results presented in Fig. (3), apparent extensional viscosity (due to only the resistance of the fluid to stretch) is calculated with Eq. (2). Results are summarized in Fig. (4). As one would expect looking at Eqns. A1 and A2, the shift in extensional viscosity depends on the shear behavior (m and n parameters) of the sample. The correction reduces the extensional viscosity measurements by roughly 20%, bringing the measurements closer to true extension.}

Figure 4: Measured (black open symbols) and corrected (blue filled symbols) extensional viscosity obtained with e-VROC^®.

SUMMARY

The significant differences in the extensional behavior of samples make accurate characterization of extensional viscosity essential for any flow process modeling. Note that for a given shear rate of 100 s^-1, the shear viscosity of Xanthan and PVDF solutions is roughly the same. However, for an equivalent extensional rate, the extensional viscosities differ by more than a factor of 2. Our results show how e-VROC^® technology is ideal for characterizing the extensional viscosity of complex fluids. Excluding the shear contribution to the measured apparent extensional viscosity through the protocol presented in this technical note is an important step toward precise characterization of the true extensional viscosity of samples.

References

[1]	T. J. Ober, "Microfluidic extensional rheometry usin a hyperbolic contraction geometry," Rheologica Acta, 2013.
[2]	"Additional details on m-VROC® technology and applications are available at www.RheoSense.com".

 

Appendix

Viscous pressure drops are obtained for power law fluids under the 2D lubrication approximation [1]:

A1. Non-Newtonian fluid:

A2. Newtonian fluid:

A3. Pressure drop components:

To read more or view additional extensional application notes, visit RheoSense.com